Sept. 30, 2014 - Convert Fractions to Decimals to Percents

Tuesday, we added one more conversion, convert fractions to decimals and then to percents.  So what is a percent?  Percent is a comparison to 100.  We have already learned to convert a fraction to a decimal, divide the numerator by the denominator (top in bottom out).  To convert a terminating decimal to a fraction you say it correctly using place value and a repeating decimal you do the "9-Thing."

To convert a decimal to a percent you just move the decimal 2 places to the right and add a percent sign.

examples:
0.25 = 25%
1.5 = 150%

To convert a percent to a decimal you move the decimal 2 places to the left and take off the percent sign.

examples:
46% = 0.46
125% = 1.25

The hardest thing to remember is which way you move the decimal.  We think about Dr. Pepper to helps us remember.  Dr. Pepper means to change a decimal to a percent you move the decimal to the right D to P.  To convert a percent to a decimal you move the decimal to the left which is P to D.  You always move the decimal 2 places because there are 2 zeros on 100.

Students will have to give the missing values when given either the fraction, the decimal, or the percent.

    Fraction         Decimal            Percent   
      3/4                  .75                   75%  

     1  65/100        1.65                  165%  
     1  13/20  


        4/5                 0.8                   80%

Sept. 29, 2014 - Convert Decimals to Fractions

Monday, we learned to convert terminating and repeating decimals into fractions or mixed numbers.  First, let's have some definitions.

terminating decimals - decimals that stop. They do not repeat.  When dividing you have a remainder of 0.
examples:  0.25,  3.674

repeating decimals - decimals that repeat a number or series of numbers continually. To write a repeating decimal, you write the number or series of numbers that repeat one time and put a bar on top of the numbers that repeat.
                   __      __
examples:  0.5,   4.45

To convert a terminating decimal to a fraction you just say the decimal correctly using place value.

examples:
0.6 = 6/10 = 3/5
4.75 = 4  75/100 = 4  3/4

To convert a repeating decimal, where the entire decimal is repeating, you do what we call the "9-thing."
You put the decimal as the numerator and for each digit that is repeating in the decimal you put a 9 in the denominator.

examples:
  __
0.5 =    5/9
   __
0.63 =  63/99 = 7/11
   ___
5.363 = 5  363/999 = 5  121/333

Week of Sept. 29th

This week we will begin discussing percents.

Monday - We will change continue with last weeks information of converting fractions to decimals and convert terminating and repeating decimals where the entire decimal is repeating.

Tuesday - We will introduce percents and convert our fractions and decimals to percents.

Wednesday - We will learn to calculate percent of a number focusing on sales tax, tip, and discount.

Thursday and Friday - We will begin solving simple 1-step equations with addition, subtraction, multiplication, and division.

Bonus Question of the Week:

On a piece of paper, copy this problem down and work it.  Make sure you show all your work and that your answer has a correct label.  Turn it in by Thursday, Oct. 2nd for 10 Bonus points.

Ryan transfers 15% of his monthly pay into a savings account. If Max makes $1850 per month, how much will he save in a year?

Wednesday, Sept. 24, 2014

Today, we will change fractions to terminating and repeating decimals.  To change a fraction to a decimal you divide the numerator by the denominator.

                       .  6

                   _____

ex.  3/5 = 5 | 3 . 0            so 3/5 = 0.6

                   -3   0

                  ______

                          0

The .6 is a terminating decimal because it stops with a remainder of 0.

A repeating decimal is a decimal number that repeats a single digit or group of digits.

                   __

ex.  1/3 =  0.3

When you divide 1 by 3 the 3 repeats.  Once it repeats 3 times you can consider it to be a repeating decimal.  You write the number of group of numbers one time and put a bar on top of the number or group of numbers that repeat.

We will also receive in class a list of 41 fraction to decimal equivalents to divide.

Click here for a list of equivalents that I want you to memorize.

We will also introduce the calculator to help with converting fractions to decimals.

Week of Sept. 22nd

This week we will have our second test, Test 1-2.  This test will cover operations with integers, mixed numbers, and decimals.  We will review on Monday and then test on Tuesday.  We are also supposed to have our first Pep Rally on Tuesday which will possibly modify our afternoon class schedule.  If I feel the students will not have enough time to complete the test I may postpone it until Wednesday.  If that happens I will just flip Tuesday and Wednesday's schedule on my calendar.

Wednesday, we will convert fractions to terminating and repeating decimals.  The students will enjoy this day because we will also introduce the graphing calculators.  The students will also receive a list of 41 fraction to decimal equivalents that I will want them to memorize.  Don't worry, there is a trick to memorizing them.

Thursday, we will carry our fractions to decimals one step further, to percents.

Friday, we will begin finding percent of a number.  We will be looking at sales tax and discount to teach this lesson.  So next time you go shopping have your child figure what the sales tax might be on your purchase.

Bonus Question of the week:

The Rodriguez family went to dinner at Pasta Palace. Mr. Rodriguez ordered a meal for
$6.25; Mrs. R ordered a meal for $7.50; the 2 children ordered individual pizzas for
$4.99 each. The sales tax rate was 7.25% and they left a 15% tip. How much was the
total bill?

Copy the question, solve, and turn it in by Friday for 10 Bonus points.

Decimal Operations

Thursday and Friday we discussed decimal operations.  This is something that the students should be good at because they have been learning decimals since 4th and 5th grade.

Addition and Subtraction

-There is one thing to remember when adding and subtracting decimals:  line up the decimals.  Along with that add zeros as place holders and add or subtract you numbers.

-To multiply decimals you multiply the numbers as though the numbers do not have decimals.  Just line up your numbers and multiply.  To determine where the decimal goes you count the numbers to the right of the decimal in each number in the problem.  The sum is equal to the number of places you move the decimal to the left in your answer.

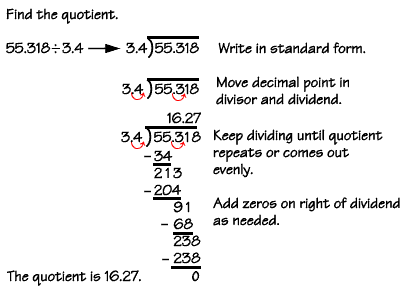
-To divide decimals you have to make sure your divisor (the number on the outside) is a whole number.  If it is not you move the decimal to the right until it is a whole number.  The number of places you moved the decimal in your divisor you move the decimal in your dividend, the number under the "house."  Once you have moved the decimal in both the divisor and dividend you bring the decimal to the top and divide.

(Images taken from Google Images)

Divide Fractions and Mixed Numbers

To divide fractions we first need to understand a reciprocal.  Reciprocals are two fractions, that when multiplied, equal 1.

Examples:

Fractions                 Reciprocals

     5 = 5/1                   1/5

     3/4                         4/3 = 1  1/3

     3  2/3 = 11/3          3/11

So you turn the number into a fraction if it is not already and then just flip it.

To help us remember how to divide fractions we learned a a little rap.

  To De-vide fractions

  Don't ask why

  Just flip the second

  And Multiply

  To Multiply fractions

  You know you've gottem

  It's top times top

  And Bottom times bottom.

If you would like to listen to the rap click here to here my class.

Here is an example of 

Multiplying Mixed Numbers

The past two days we have been learning to multiply positive and negative mixed numbers.  The students found this to be easier than expected.

To multiply mixed numbers you first must change each number in the problem to a fraction.  If you have a mixed number you use the "Popcorn Method" to change it to a fraction.

If the number is a whole number you just put a 1 as the denominator.  Once you have fractions you multiply the numerators together and then the denominators and simplify your answer.

In this example we simplified our answer by dividing 195 by 20.  The second way we simplified we looked for what 15 and 20 had in common and divided by the common factor.  The students learned that there is a 3rd way to simplify.  You can simplify the problem before you multiply.

Week of September 15th

This week in class we will finish mixed number operations and begin decimal operations.  The students did very well adding and subtracting positive and negative mixed numbers.  I was very pleased with how they performed.

Students will receive their 1st six weeks Progress Reports on Tuesday.  I realize some parents are used to the Parent Portal from the previous gradebook, hopefully, it will be available soon.

Math and Science teacher's will be trained on our new digital textbooks this next week.  So students should be getting information of the books soon as well.

Wednesday is Fall Picture day.

Bonus Problem for Students - worth 10 points

"The training session held 50 people. On Monday 38 people attended. On Wednesday 46  people attended. On Thursday only 1/2 as many as Wednesday's group attended. How many  people attended this week?"

To earn the 10 points you must write the question of a sheet of paper, answer the question with its correct label, and turn it in by Friday, Sept. 19th.

Add and Subtract Mixed Numbers

Wednesday we began adding and subtracting mixed numbers with a slight twist, negatives.  We began class by discussing what a fraction looks like.  I had the students draw a picture of 5/8. I noticed that the students, for the most part, understand the true meaning of what a fraction is.  Here are the 2 correct answers out of 3 that they gave me:

A fraction is a part to total relationship.  5/8 means it takes 8 pieces to make a whole and I have 5 of them.

Next, we review the steps to add and subtract fractions.

1.  Find a common denominator
2.  Change your numerator to match
3.  Add or subtract your numerators
4.  Keep your new denominator
5. SIMPLIFY!!!!!

Then we moved to mixed numbers.  We added one step when adding or subtracting mixed numbers and that was to add or subtract your whole parts.  I like putting that step between step 4 and 5.

At this point I told the students we are finally ready to begin our lesson which was to add or subtract mixed numbers with negatives.  We did this problem:

The students realized that you answer this question just like any other you just have to throw in your integer rules.

We will finish this lesson on Thursday.

Integer 4-Corner Model

Today, we began a small project.  Students are to create a 4-Corner Model with their integer rules.

In each section the students are to:

- Title the section (ex. Add Integers),

- In complete sentences, provide the rules to complete the operation,

- Give 2 examples of the operation.

In the center section, the students are to write a word problem using one of the operations.  The problem does not have to unique.  They can copy one from a previous assignment, find one in a textbook, or create one themselves.

Students can make this 4-Corner Model using paper like the image above, or they may choose their own medium, such as a Google Doc, Google Presentation, a Prezi, a Smore, etc. whatever they choose.

This project is due, if a paper copy, by the end of the day Thursday, Sept. 11.  If the project is digital they have until midnight Thursday.

Divide Integers

Today, we discussed the rules for division of integers.  The students found out that the rules for division are the same as the rules for multiplication.

There are 2 steps for dividing integers:
Step 1:  Divide your numbers
Step 2:  Count your negatives
   - If you have an even number of negatives your answer will be positive.
   - If you have an odd number of negatives your answer will be negative.

Examples:

24 / -4 = -6            -32 / -8 = 4

Tomorrow we will be working on a small project to help us better understand our integer rules.  Students will be able to create their project with regular paper, or they can create a digital presentation using Google, or a Prezi, or a Smore, etc.  More information will be provided in class tomorrow.

Week of Sept. 8 - 12

This week we will finish our lessons on Integers.  We will discuss division of integers on Monday.  We will find out that the rules for division will be the same as the rules for multiplication.  We will also get our test back from Friday.  The results were not bad but there is still much work to be done.

Tuesday, we will begin a small project to help us review the integer rules.

Wednesday, the students will be happy that we will not have anymore lessons on Integers but they will find our that the rules will still apply to positive and negative fractions and decimals.  We call these Rational Numbers.  So Wednesday, we will discuss add and subtracting fractions and mixed numbers.  This should be a review.  We will see.

Thursday, we will examine subtraction of mixed numbers where you have to borrow from the first mixed number.

Friday, we will begin a 2 day lesson on multiplication of mixed numbers.

Friday will also be the end of the first 3 week period of school.  Expect to see a progress report first of next week.

Test 1-1 Review

Today in class we completed a Test Review because tomorrow we will be taking our first test of the 2014-2015 school year.

The topics include:
-Writing an integer to represent a situation.
-Comparing and ordering a list of integers.
-Absolute value of a number
-Add, Subtract, and Multiply Integers

The students should have a copy of the test review with the correct answers, but if not here is a link to a blank review and here is a link to the answers to the review.

This question always comes up just before the first test of the year, "How do I study for a math test?"
Here are a couple of ideas:
- Print the review and try to work all the problems again.
- Read over your notes so that you can remember what they say without having to look at them.
- If there are rules to learn be able to quote them and apply them.
- Look back over old assignments. Work some of the problems we did not complete.

There are many ways to study besides these but this is a start.

Good luck on your test tomorrow.

Multiply Integers

Today we discussed the rules for multiplying integers.  We found out that to multiply integers we use two basic steps.

Step 1:  Multiply the numbers like you have in the past.  Pretend the numbers are all positive.

Step 2:  Count the number of negative signs in the problem.
     - If you have an even number of negative signs your answer will be a positive.
     - If you have an odd number of negative signs your answer will be a negative.

Examples:

6 x 3 = 18

-5 x 3 = -15        There is 1 negative so the answer is negative.

-7 x -4 = 28         There is 1 negative so the answer is negative.

-2 x 3 x -2 = 12          There are 2 negatives so the answer is positive.

-5 x -2 x -2 = -20        There are 3 negatives so your answer is negative.

-1 x -1 x -1 x -1 x -1 x -1 x -1 = -1         There are 7 negative signs so the answer is negative.

If you need additional instruction click here.

Subtract Integers

Today we discussed the procedure to subtract integers.  Ex.  15 - (-21) =  We determined that to subtract integers you really have to add.

We use a process of LCO+ to work a subtraction of integers problem.  LCO+ stands for:

L - leave the first number alone
C - change the subtraction sign to an addition sign
O - change the next number, after the subtraction sign, to its opposite
+ - revert back to your addition rules because the problem is now addition

Ex.  15 - (-21) =

       15 + 21 = 36

The 15 stayed the same.  We changed the - sign to +, and the 21 changed from negative to positive.  The signs of the new problem are alike so we add them together.

Additional examples:

Ex.   -6 - (-8) =                               -9 - 7 =

        -6 + 8 =  2                              -9 + (-7) = -16